Saturday, March 31, 2012
7-1
Sunday, March 25, 2012
Review of Probability
Ex 1: A standard deck of cards consists of 52 cards, with 13 cards in each of four suits (clubs, spades, diamonds, and hearts). Clubs and spades are black, and diamonds and hearts are red. The face cards consist of jacks, queens, and kings. Assuming the deck is shuffled properly, what would be the probability that a card is:
- red = 26/52 = 1/2
- A black club = 13/52 =1/4
- blue = 0/52 = 0
- A jack = 4/52 = 1/13
-Sameer
review of matrices,WOOHOO
-A^t means transpose. That simply means switch the rows and columns.
-To add matrices they must be the same dimensions (number of rows and columns)
-To complete scalar multiplication you multiply every entry by the number.
EXAMPlE TIIIIIIIIIIIIME
M= [7 1 5]
[4 3 2]
A. What are the dimensions of the matrix?
2x3 ( There are two rows and three columns )
B. Find m^t
[4 7]
[3 1]
[2 5]
Example TWO:
Add the matrix from the last example and the matrix
[8 3 4]
[6 2 9]
Simply add the corresponding spots.
[7+8 1+3 5+4]
[4+6 3+2 2+9]
The answer would then be:
[15 4 9]
[10 5 11]
So that is how you work with matrices.
--Sarah (:
MORE BLOGS YAY
1) take the log or ln of both sides
*use ln where there is an e*
2) put into calculator if asked exact values don't get input in a
calculator
EX:
4^x=16
log4^x=log 16
xlog4=log16
x= log16/log4
x=2
exponents that are variables
1) take the log or ln of both sides *use ln where there is an e*
2) put into calculator if asked exact values don't get input in a calculator
EX:
3^x = 12
log 3x = log 12
x= log 12 / log 3
x= 2.26
(the square root of) e^x = 50
ln e^1/2x = ln 50
x= (ln 50 / ln e) *2
x=7.82
Cos(a +/- B) and sin(a +/- B)
- cos(a +/- B) = cosa(cosB) -/+ sina(sinB)
- sin(a +/- B) = sina(cosB) +/- cosa(sinB)
- sinx + siny = 2sin(x + y/2) cos(x - y/2)
- sinx - siny = 2cos(x + y/2) sin(x - y/2)
- cosx + cosy = 2cos(x + y/2) cos(x - y/2)
- cosx - cosy = -2sin(x + y/2) sin(x - y/2)
Example 1: Find the exact value of cos75 degrees.
- You will first go to your trig chart to find out what adds to give you 75 for cos.
- Those numbers are 45 and 30.
- You are going to use those numbers in the first formula from above.
- cos(45 + 30) = cos45(cos30) - sin45(sin45)
- Now you plug in what those are from the trig chart.
- (square root of 2/2)(square root of 3/2) - (1/2)(square root of 2/2)
- square root of 6/4 - square root of 2/4
- Final answer: square root of 6 - square root of 2/4
Example 2: sin30(cos15) + cos30(sin15)
- For this problem they give you the two numbers they are adding so you have to replace it with one of the formulas.
- If you look at the problem and the formulas you have from above, it is the same as sin(a + B).
- Now you can replace.
- sin(30 + 15) = sin(45)
- Now that you have gotten an answer, you have to see what sin(45) is on the trig chart.
- sin(45) on the trig chart is square root of 2/2.
- Final answer: square root of 2/2.
-Amber :)
Review of Trig Identities
Notes:
Reciprocal
• csc (theta)=1/sin (theta)
• sec (theta)=1/cos (theta)
• cot (theta)=1/tan (theta)
• tan (theta)=sin (theta)/ cos (theta)
• tan (theta)=cos (theta)/ sin (theta)
Pythagorean
• sin^2 (theta) + cos^2(theta) = 1
• 1 + tan^2 (theta) = sec^2 (theta)
• 1 + cot^2 (theta) = csc^2 (theta)
Cofunction
• sin(theta) = cos (90 degrees-theta)
• cos (theta) = sin (90 degrees-theta)
• tan(theta) = cot(90 degrees-theta)
• cot (theta) = tan (90 degrees-theta)
• sec (theta) = csc (90 degrees-theta)
• csc(theta) = sec (90 degrees-theta)
Example: Simplify: (sec^2x – tan^2x)/cos x
• You would use the identity that contains tan^2 and sec^2
• That would give you 1/cos x
• Your final answer would be secx
-Braxton-
Trig Reviewww
This week, I'm going to do some review from one of the trigonometry sections. If you do remember any trig, you should be good to go and could probably work my example right now. But incase you don’t remember everything, i'm going to give you some information. all you need to know right now in trig is basic formulas and the trig chart. For starters, you must remember:
- Tan=sin/cos
- Sin=1/csc
- Sec=1/cos
- Cos=1/sec
- Tan=1/cot
- Cot=1/tan
- Csc=1/sin
Apart from knowing that, you must also know the Pythagorean Identities. To refresh your memories, these are the Identities you will need to use when doing a lot of trigonometry.
- 1+tan^2=sec^2
- 1+cot^2=csc^2
- Sin^2+cos^2=1
Now that we’ve went over some of the basic things needed to work the examples I am about to show you, we can begin!!
Example 1: Simplify:
(Sinx^2+Cosx^2)/ Sinx
- Look for identities. You have one, so simplify it.
- You now get 1/Sinx. You can simplify this by referring to the notes above.
- You get Cscx as your final answer J
Saturday, March 24, 2012
Using formulas cos(a +- B) and sin (a +- B)
- B) and sin(a+ or - B). There are 6 different formulas for these types of problems. You should already know these formulas because I have already went over these types of problems, but to refresh you memory I will list them again.
The formulas are:
- cos(a + or - B)=cosa cosB - or + sina sinB
- sin(a + or - B)=sina cosB + or - cosa sinB
- sinx+siny=2sin (x+y/2) cos (x-y/2)
- sinx-siny=2cos (x+y/2) sin (x-y/2)
- cosx+cosy=2cos (x+y/2) cos (x-y/2)
- cosx-cosy=-2sin (x+y/2) sin (x-y/2)
Now that you should remember the formulas, I am going to work a few examples which should help you to understand how to work these problems. They are very simple as long as you know the formulas above.
Example 1: cos 105 degrees
- You are going to use your trig chart to help work this problem.
- You are going to use formula 1 to solve this problem.
- Since 45 and 60 degrees are on the trig chart and they add up to equal 105, you are going to use those two degrees.
- cos(45+60)=cos45 cos60-sin45 sin60
- You then plug those into the trig chart.
- You answer is going to be: square root of 2 - square root of 6/4
Example 2: sin75 cos15 + cos75 sin15
- You are going to replace this with one of your formulas above.
- That formula above is the same as sin(a+B)
- Once you replace with that formula you are going to get sin(75+15)=90 degrees
- sin 90 degrees on the trig chart equal 1
- 1 is goin to be your answer
And that is how you work problems using formuals for cos(a+B) and sin(a+B). Well that is is for this weeek. Byeeeee.
--Halie!
Thursday, March 22, 2012
5-7
- Take the log or ln of both sides. Use ln when the number is e.
- Put into your calculator only if asked to. Exact values do not get input into a calculator.
Monday, March 19, 2012
Logsssss!
y = logb^x is equivalent to x = b^y
where b is the common base of the exponential and the logarithm.
The forms above helps in solving logarithmic and exponential functions and needs a deep understanding. Examples, of how the above relationship between the logarithm and exponential may be used to transform expressions, are presented below.
Example 1 : Change each logarithmic expression to an exponential expression.
1. log3^27 = 3
2. log36^6 = 1 / 2
3. log2^(1 / 8) = -3
4. log8^2 = 1 / 3
Solution to Example 1:
1. The logarithmic form log3^27 = 3 is equivalent to the exponential form
27 = 33
2. The logarithmic form log36^6 = 1 / 2 is equivalent to the exponential form
6 = 361/2
3. log2^(1 / 8) = -3 in exponential form is given by
1 / 8 = 2-3
4. log8^2 = 1 / 3 in exponential form is given by
2 = 81/3
logs
EX:put in exponential form
log base 4 16 = 2
you take the base which is 4 and you raise is to what the whole thing equals
4^2 = 16
expand:
log (MN)^2
when you expand logs you put in terms of addition if they are multiplied and subtraction if they are divided and you put exponents in front of the equation
2 log M + 2 log N
condense:
log 8 + log 5 - log 4
to condense you just do the expanding steps backwards.
log (8)(5) / 4
log 40/4
log 10
since this is log 10 the base i also 10 which cancles everything and your equation equals 1
Blog STuff YAY
Notes from class
- Logs can be written as exponents and it works the other way
- Convert to exponential form to solve a log
- If no base is there than it is 10
- For natural log, no base is implied to be e
- Shortcut: If b and x are equal, they cancel and leave you with exponent
Example
solve the log
log(base 2) 4 = x
2^x=4
x=2
YAY!
Sunday, March 18, 2012
Logs
Notes:
• One thing to remember is if the base of the log isn't there then it is assumed that the base is 10.
• Another thing is logs can be written as exponents and vice versa for example loga=10, it is implied that log base 10 raised to the tenth power is a.
• here's a shortcut: If b and x are equal, then they cancel out and the exponent left will be your answer
Ex 1: log (base 3) 81=x
• When solving for x you get 4 since 3^4 = 81
• Your final answer will be 4
Alright, so here are the things you will need to know:
• Logs can be written as exponents and vice versa. For example: log x = a could also be written as 10^a = x. 10^c = 4 could also be written as log 4 = c
• Whenever you solve a log, you have to convert it to exponential form
• If the base of the log is not written, it is implied that the base is 10
• If the base of the natural log is not written, it is implied that the base is e
• Shortcut: If b and x are equal, then they cancel out and leave you with the exponent as your answer
EXAMPLE:
Rewrite the following in exponential form then solve.
Log28=3
Remember, base^right side of equal sign=left side of equal sign
2^3=8
THAT IS ALL.
--Sarah(:
Alright, so here are the things you will need to know:
• Logs can be written as exponents and vice versa. For example: log x = a could also be written as 10^a = x. 10^c = 4 could also be written as log 4 = c
• Whenever you solve a log, you have to convert it to exponential form
• If the base of the log is not written, it is implied that the base is 10
• If the base of the natural log is not written, it is implied that the base is e
• Shortcut: If b and x are equal, then they cancel out and leave you with the exponent as your answer
EXAMPLE:
Rewrite the following in exponential form then solve.
Log28=3
Remember, base^right side of equal sign=left side of equal sign
2^3=8
THAT IS ALL.
--Sarah(:
5-3
Notes:
• Logs can be written as exponents and vice versa. For example: log x = a could also be written as 10^a = x. 10^c = 4 could also be written as log 4 = c
• Whenever you solve a log, you have to convert it to exponential form
• If the base of the log is not written, it is implied that the base is 10
• If the base of the natural log is not written, it is implied that the base is e
• Shortcut: If b and x are equal, then they cancel out and leave you with the exponent as your answer
Example: log 1000 = x
• 10^x = 1000
• When you solve for x you get 3 because 10^3 = 1000
• Your final answer would be 3
-Braxton-
5-3 Logarithms
Notes:
- Logs are another way to write exponents. For example, logb X=a as an exponent is b^a=x/
- To solve a log, you write as an exponent.
- If no base is written it's implied to be 10.
- The base of ln is always e.
- If b and x are equal they cancel along with the log, leaving the exponent as your answer.
Okay, so now that you know a few basic things about logarithms, I am going to work a few example for you.
Example 1: log 100 = x
- Since there is no base number, you automatically assume that the base is 10.
- You then switch it around and get 10^x=100
- Once you do that, you solve for x and you end up with 2.
Example 2: ln x=-1.5
- Since there is no base number, you automatically assume that the base is e.
- You should know that because of the notes I gave you above.
- You then switch is around just like you did in example one and get e^-1.5=x
- You then have to solve for x and you should end up with x=.22 as your answer.
And that is how you work these types of problems. They are very simple and should be easy to learn. Well that is it for this weeek. byeee.
--Halie!
Saturday, March 17, 2012
5-6
- logb MN = logb M + logb N
- logb M/N = logb M - logb N
- logb M = logb N if and only M = N
- logb MO^k = klogb M, for any real number k
Sunday, March 11, 2012
This will only work if the infinite sum of a geometric series if r < 1
The formula for finding the infinite sum of a geometric series is:
S= ((t1) / (1-r))
To find where an infinite geometric converges, set r < 1 and solve for the x term
this how you are to write a repeating decimal as a fraction. To do this follow this formula: (what’s repeating / place-1)
Ex 1: Find the sum of the infinite geometric series: -3, 12, -36, 144...
• S=t1 / 1-r = -3 / 1- (-4)
• S=24 / 5
-Sameer
Infinite Sums
Notes
• You can only find the infinite sum of a geometric series, it will not work for an arithmetic series
• You can only find the infinite sum of a geometric series if r < 1
• The formula for finding the infinite sum of a geometric series is as follows:S= ((t1) / (1-r))
• To find where an infinite geometric converges, set r < 1 and solve for x
• how to write a repeating decimal as a fraction. To do this follow this formula: (whats repeating / place-1)
Example
Find the sum of the infinite geometric series.
6 + 12 + 24…
r= 12/6= 2 24/12=2
solve
6/1-2=6/-1= -6
YAY!! i did a blog
13-5
First things first, let’s see those notes!
• You can only find the infinite sum of a geometric series, it will not work for an arithmetic series
• You can only find the infinite sum of a geometric series if r < 1
• The formula for finding the infinite sum of a geometric series is as follows:
S= ((t1) / (1-r))
• To find where an infinite geometric converges, set r < 1 and solve for x
• Another thing that you need to know about this section is how to write a repeating decimal as a fraction. To do this follow this formula: (what’s repeating / place-1)
Alright how about an example?
Find the sum of the infinite geometric series.
9-6+4-…
First find r. -6/9= -2/3, 4/-6= -2/3
Next plug into the formula
S= 9 (first term)/1- (-2/3) (that’s r)
S= 9/ 5/3= 27/5
That’s all there is to it guys.
--Sarah
13-5
Exponents
There are several rules to exponents that are not necessarily difficult if you do them in the proper order. I'm going to work the following problem to give you an idea of how many different steps a problem may take to simplify.
(3^-2 + 3^-3)^-1 (Order of Operations states to deal with the stuff inside the () first)
(1/6 + 1/9)^-1 (Because the exponents were negative I took the recipricol and dealt with the exponent in the denomenator. After that add the fractions.)
(5/18)^-1 (Because there is a negative exponent on the outside, of the parenthesis you will take the recipricol of the fraction.)
(18/5) = Answer
~ Parrish
13-5
- You can not find an infinite sum for an arithmetic series.
- Geometric series where |r| < 1 are the only series that have an infinite sum.
- Formula: S = t1/1-r
- Set |r| < 1 and solve for x when you are finding infinite geometric converges.
- When you are writing a repeating decimal as a fraction you do this formula: repeating/place - 1.
Example 1: What is the fraction form of this decimal? .36363636
- First you have to find the number that is repeating: 36
- Now you follow the formula: repeating/place -1
- 36/100-1 (you put 100 because the first 36 is in the 100th place)
- You would then end up with 36/99
- Final answer: 36/99
Example 2: What is the sum of the infinite geometric series: 36 - 12 + 4 - . .
- First you must know that from the formula in the above notes, S stands for the sum.
- T1 is the first term that you are given in the series which is 36
- R is the number that is being multiplied to get to the next number which is -3. It is- 3 because 36/-12 = 3 and -12/4 = -3.
- Now you can plug the numbers into the formula.
- Formula: S = t1/1 - r.
- S = 36/1 - (.3) = 36/4 = 9
- Final answer: S = 9
- Amber :)
13-5
Notes:
• You can only find the infinite sum of a geometric series, it will not work for an arithmetic series
• You can only find the infinite sum of a geometric series if r < 1
• The formula for finding the infinite sum of a geometric series is as follows:
S= ((t1) / (1-r))
• To find where an infinite geometric converges, set r < 1 and solve for x
• Another thing that you need to know about this section is how to write a repeating decimal as a fraction. To do this follow this formula: (what’s repeating / place-1)
Example: Find the sum of the infinite geometric series: 24-12+6-3
• S=t1 / 1-r = 24 / 1- (-1/2)
• S=24 / (3/2)
• S=16
-Braxton-
exponents
these are just some common notes that will be used in the examples below.
EX:
1) (-4)^-2
-1/4 *-1/4
= 1/16
2) -4^-2
=-1/16
3)(5*2)^-3
5^-3 * 2^-3
=1/125 * 1/8= 1/1000
EXPONENTS!
| Law | Example |
|---|---|
| x1 = x | 61 = 6 |
| x0 = 1 | 70 = 1 |
| x-1 = 1/x | 4-1 = 1/4 |
| xmxn = xm+n | x2x3 = x2+3 = x5 |
| xm/xn = xm-n | x6/x2 = x6-2 = x4 |
| (xm)n = xmn | (x2)3 = x2×3 = x6 |
| (xy)n = xnyn | (xy)3 = x3y3 |
| (x/y)n = xn/yn | (x/y)2 = x2 / y2 |
| x-n = 1/xn | x-3 = 1/x3 |
| And the law about Fractional Exponents: | |
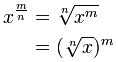 |  |
13-5
Notes:
- You can't find an infinite sum of an arithmetic series.
- Only geometric series where lrl < 1 have an infinite sum.
- The formula for these types of problems are S=t1/1-r
- When finding the infinite geometric converges, set lrl < 1 and solve for x.
- The last thing you need to know is, to write a repeating decimal as a fraction you would do what's repeating/place - 1
Okay, so now that you know all of that I am going to work a few examples for you. This should help you better understand what is going on.
Example 1: Write this repeating decimal as a fraction .23232323
- The number that is repeating is 23
- So you would put 23/100 -1
- You would put 100 - 1 becaue the 3 is in the 100ths place.
- So you would then get 23/99.
- And your answer is 23/99.
Example 2: Find the sum of the infinite geometric series.. 24-12+6-3+...
- You are going to use the formula I gave you above which is S=t1/1-r.
- S is what you are trying to find which is the sum.
- t1 is the first term given to you, which is 24
- r is the number that is being mulitplied to get the next number, which is (-1/2).
- So then you are going to plug into the formula.
- S= 24/1-(-1/2)
- Your answer is going to be S=16
Well that's it for this week. Byeeee
--Halie! :)
Friday, March 9, 2012
13-5
- S = t1/1 - r
- S = 24/1 - (-1/2)
- S = 24/1 + 1/2
- S = 16
- 1 + x^2 + x^4 + x^6 +...
- lx^2l < 1
- -1 < x^2 < 1 (square root all three terms to get your answer)
- -1 < x < 1
- 7/10-1 (the repeating decimal ends in the 10th's place)
- 7/9
Sunday, March 4, 2012
Sequences
An Arithmetic Sequence is a sequence that is characterized by adding the same number each time.
The formula for arithmetic sequences is
tn=t1 + (n-1) x d
d is what is added or the difference.
tn is __ terms
A Geometric Sequence is a sequence that is characterized by mulitplying the same number each time.
The formula for geometric is
tn=t1 x r^(n-1)
r is whatever is being mulitplied
n is the term number
tn is __ terms
Ex 1: Find the tn formula for the following sequence:
2,5,8,11
It is arithmetic since 3 is added each time. So we plug it into the tn formula.
Tn=2+(n-1)3
Tn=3n-1
Ex 2: Find the tn formula for the following sequence:
2,6,18,54
Tn=2(3)^n-1
-Sameer
13-3
-To find the sum of the ____ terms of an arithmetic series:
Sn=n (t1 + tn)/1-r
-To find the sum of the ____ terms of a geometric series.
Sn= t1 (1-rn)/1-r
-A series is a list of numbers that are added together.
2+4+6+8
EXAMPLE TIME BOYS AND GIRLS
Find the sum of the first 8 terms of the arithmetic series.
11+14+17+20+…
First of all, set up the equation
S8= 8 (11 + )/2
To find tn, use your knowledge of the previous sections. T8= 11 + (n-1) (3)
T8= 11+3n-3 = 11 + 24-3= 25-3
T8=22
Plug this number into your equation next.
S8= 8 (11 + 22)/2= 132
That is about all there is to it. Do not forget your assignments that are due tomorrow! Have a fantastic week!
--SARAH (:
13-4 infinite limits
EX: lim n --> (infinty) n+5/ n
look at all the rules
top = bottom
1/1 = 1
13-1 Arithmetic and Geometric Sequences
Notes:
• Arithmetic Sequences
o They are generated when the same number is added every time
o The formula for this type of sequence is tn = t1 + (n-1) * d
o d= what is being added, or the difference
o tn= ___ term
o t1= 1st term
o n= term number
• Geometric Sequences
o They are generated when the same number is multiplied every time
o The formula for this type of sequence is tn = t1 + r^(n-1)
o You cannot divide, so you have to multiply by fractions
o r = what is being multiplied
Example: tn= 2n + 5; find the first four terms and state whether the sequence is arithmetic or geometric
• 2 (1) + 5 = 7
• 2 (2) + 5 = 9
• 2 (3) + 5 = 11
• 2 (4) + 5 = 13
• 7, 9, 11, 13,…..
• This sequence is arithmetic; +2
-Braxton
Sequences!
An Arithmetic Sequence is a sequence that is generated by adding the same number ech time.
The formula for arithmetic is
tn=t1 + (n-1) x d
d is what is being added or the difference.
tn is __ terms
A Geometric Sequence is a sequence that is generated by mulitplying the same number each time.
The formula for geometric is
tn=t1 x r^(n-1)
r is what is being mulitplied
n is the term number
tn is again __ terms
Now that you know some information on this, we're going to work some problems.
Example 1. Find the tn formula for the following sequence:
1,4,7,10
This is arithmetic because you add 3 each time. So we plug it into the tn formula.
Tn=1+(n-1)3
Tn=3n-2
Example 2: Find the tn formula for the following sequence:
2,4,8,16
This is geometric because you multiple everything by 2.
Tn=2(2)^n-1
I hope you learned about sequences. See you next week!
--Carley!
arithmic and geometric sequences
Here are some things for arithmic
- Arithmetic Sequence is a sequence that is generated by adding the same number ech time.
- The formula for arithmetic is tn=t1 + (n-1) x d
- d= what is being added or the difference.
- tn= blank terms
- Geometric Sequence is a sequence that is generated by mulitplying the same number each time.
- The formula for geometric is tn=t1 x r^(n-1)
- r= what is being mulitplied
- n=term number
- tn=blank terms
tn=2n+2
2(1)+2=4
2(2)+2=6
2(3)+2=8
2(4)+2=10
Here is an example of a geometric sequence
tn=2x3^n
2x3^1=6
2x3^2=12
2x3^3=24
2x3^4=48
bam! all done!
13-1
13-1
- A sequence that is generated by adding the same number each time is called the arithmetic sequence.
- Formula: tn = t1 + (n-1) x d
- d = what is being adding/difference
- tn = blank terms
- t1 = first term
- n = term number
- A sequence that is generated by multiplying the same number each time is called the geometric sequence.
- Formula: tn = t1 x r^(n-1)
- r = what is being multiplied
- n = term number
- tn = blank terms
- t1 = first term
- A sequence is a list of numbers.
Example 1: tn = 6n + 20. What is the sequence? Is it geometric, arithmetic, or neither?
- First thing you need to know is you plug in the number of terms you want to find out for n.
- You are going to find out the first four terms.
- T1 = 6(1) + 20 = 26
- T2 = 6(2) + 20 = 32
- T3 = 6(3) + 20 = 38
- T4 = 6(4) + 20 = 44
- Now we have to find out what is the sequence.
- Each time you are adding 6 to get to the next number so that means it is an arithmetic sequence.
Example 2: tn = 5 x 10^n-1. What is the sequence? Is it geometric, arithmetic, or neither?
- Just like in example one, you have to plug in the number of terms you want to find our for n.
- You are going to find the first four terms again.
- T1 = 5 x 10^1-1 = 5 x 10^0 = 5
- T2 = 5 x 10^2-1 = 5 x 10^1 = 50
- T3 = 5 x 10^3-1 = 5 x 10^2 = 500
- T4 = 5 x 10^4-1 = 5 x 10^3 = 5000
- Now you have to find out the sequence.
- Each time you are multiplying 10 to get to the next number so that means it is an geometric sequence.
- Amber :)
Saturday, March 3, 2012
13-1
- arithmetic sequence: a sequence that is generated by adding the same number each time
- geometric sequence: a sequence that's generated by multiplying the same number each time *to divide, we use fractions (/2 = x 1/2)
13-1 Arithmetic and Geometric Sequences
Notes:
- Arithmetic Sequence is a sequence that is generated by adding the same number ech time.
- The formula for arithmetic is tn=t1 + (n-1) x d
- d= what is being added or the difference.
- tn= blank terms
- Geometric Sequence is a sequence that is generated by mulitplying the same number each time.
- The formula for geometric is tn=t1 x r^(n-1)
- r= what is being mulitplied
- n=term number
- tn=blank terms
A sequence is a list of numbers.
Okay, so now that you know all what you need to, I am going to work a few examples for you.
Example 1: tn=2n + 3. Figure out the sequence and if it is geometric, arithmetic, or neither.
- You would plug in the number of terms for n.
- 2(1) + 3 = 5
- 2(2) + 3 = 7
- 2(3) + 3 = 9
- 2(4) + 3 = 11
- From that you can tell that the sequence is arithmetic because it is adding 2 to get to the next number.
Example 2: tn=3 x 2^n. Figure out the sequence and if it is geometric, arithmetic, or neither.
- You would do the same thing as in example one.
- 3 x 2^(1)= 6
- 3 x 2^(2)= 12
- 3 x 2^(3)= 24
- 3 x 2^(4)= 48
- From that you can tell that the sequence is geometric because it is mulitiplying 2 to get the next number.
So that is how you work those types of problem. Byeee.
--Halie !